Enchanting Instruments 70 - Electronic Sound Source Mechanism 10: Additive Synthesis

Periodic sound can be expressed by synthesizing sine waves. Additive synthesis is based on this theory and it creates a sound with a sense of pitch by adding arbitrary integer multiples of sine waves.

I would like to mention that the concept of pipe organ is additive synthesis, although it is not electronic. Mechanical Hammond organs also inherit this concept.

Yokohama Minatomirai Hall Pipe Organ “Lucy”, Public domain (Source: Wikipedia)

As shown in the photo below, pipe organs create sound by pulling and pushing the stop levers on either side of the keyboard to select the pipes to be played.

Weingarten Basilika Gabler-Orgel Spieltisch, CC BY 3.0 (Source: Wikipedia)

Next is a mechanical Hammond organ that imitates a pipe organ. The tone wheel is turned electronically, which is then converted into an electrical signal by a pickup to produce an electronic sound.

Hammond b3, CC BY-SA 3.0 (Source: Wikipedia)

Mechanical Hammond organs have a lever on the keyboard called a drawbar that creates sound based on the principle of adding harmonics.

Hammond b2, CC BY-SA 2.0 (cited from Wikipedia)

Let’s take a look at the drawbar. The number on the front of the drawbar shows the length of the pipes, which is the idea of combining nine foot pipes to create a sound, which is actually an overtone to the note that is being played. At the time, this was an alternative to pipe organs, so I think it’s easier to visualize the length of the pipes than to write the overtone values. Also, numbers 1-8 written directly on the drawbar correspond to the volume. The maximum number you can see is the current volume.

The third white lever from the left, the drawbar marked 8 feet (2.4384m), is the bass note and is the note that’s being played.

The reddish-brown lever on the far left is marked 16 feet (4.8768m), which means it is twice as long as the bass note. This means that you can play an octave below the note you are playing.

The second reddish-brown lever from the left is to the left of the fundamental, but it is marked 5 1/3 (1.6256m), which is shorter than the fundamental. This makes it 5 degrees above the bass note. The reason it is to the left of the main note is because it is not an overtone of the main note, it is a third overtone of 16 feet, and to the right of the main note is an overtone.

The octave-related levers are white, and the other levers are black. However, there is no 7th overtone.

• 4: White 2 overtone (1 octave)
• 2 2/3: Black 3 overtone
• 2: White 4 overtone (2 octaves up)
• 1 3/5: Black 5 overtone
• 1 1/3: Black 6 overtone
• 1: White 8 overtone (3 octaves up)

The video below shows the movement of the frequency spectrum when A4 (440 Hz) is played and each drawbar is pulled out. The sound of each bar is almost a sine wave, corresponding to an overtone. The combination of these controls the tone. Even when all are maximized, the levels are quite varied.

The drawbars create tones, but the process is additive synthesis itself. At first glance, the number of overtones that can be controlled may seem small, but the closer the overtones are to the base tone, the greater their effect on the tone, so this may be about the right amount for people to handle sensitively. If there were a hundred of these levers, most people would be confused, and it would be impossible to control the tone instantly.

Additive synthesis has been done in electronic instruments since the early days. With the use of computers, even higher harmonics can be handled. However, although an increase in the number of overtones allows for greater tonal flexibility, it is not suitable for intuitive sound creation. For this reason, synthesizers that use only additive synthesis to create sounds are rarely seen.

1980's alpha Synthauri Corporation

Below is a screenshot of the softsynth Audio Damage Phosphor, a reproduction from the early 1980s of a system running on an Apple II computer called alpha Synthauri Corporation.

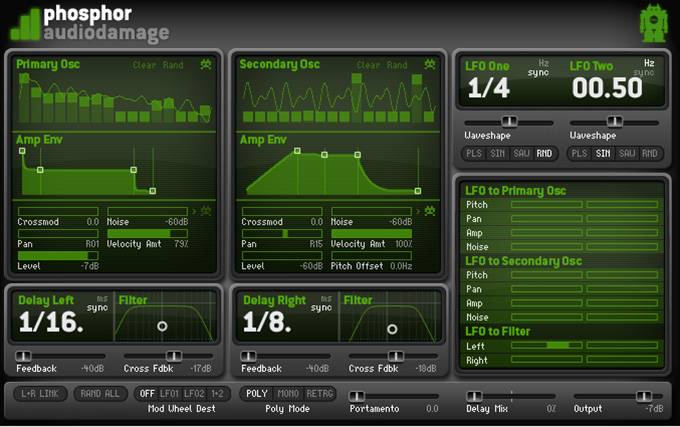
It has two oscillators that can control up to 16 harmonics. Since you can use two sets of 16th overtones, twice as many as the Hammond organ, you can create your own tones quite freely. As you can see when you actually use this software, the variation of tones is as good as analog synthesizers. However, it is digitally straight-forward and generates fold-back noise. Perhaps the actual machine was made that way.

KAWAI K5000

kawai k5000, CC BY 2.0 (Source: Wikipedia)

Calculating all overtones requires processing power, so additive synthesizers that appeared in the 1980s were designed to reduce calculation costs by synthesizing them beforehand. Although not made today, Kawai synthesizers of the 1980s and 1990s were based on additive synthesis. Among them, the flagship model K5000 is a full-fledged additive synthesizer capable of synthesizing up to 64 overtones. However, since it is difficult to create sounds by directly manipulating the overtones, various innovations have been made.

Additive synthesis is difficult to learn and control, so it is not often seen. Nevertheless, there are some soft synths that can also handle additive synthesis.

u-he Zebralette Free

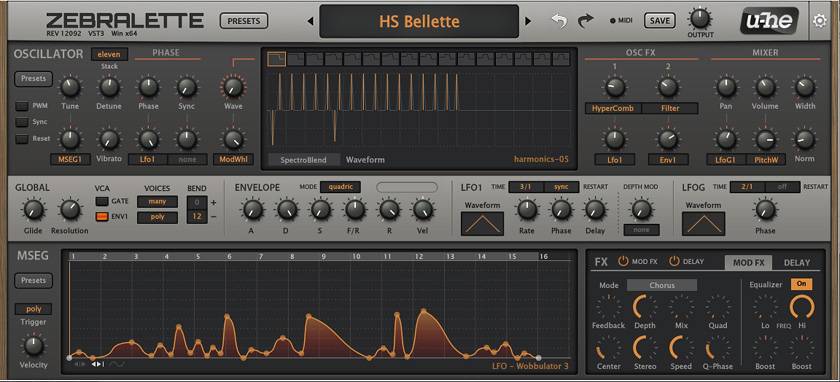
Zebralette has an additive synthesis mode for the oscillator. It can handle up to 128 overtones plus or minus, and it doesn’t generate fold-back noise. However, it is difficult to create sounds with this number of controllers, so usually, sounds are created in a separate mode. Personally, I use it only when I want to play a few specific overtones. Even so, it is quite a hassle to check how many overtones are being played.

Difficulties in creating additive synthesis sounds

If the number of overtones is about the same as a pipe organ, it is possible to grasp the relationship between the imagined sound and the overtones, but with 128 overtones like the Zebralette, it’s necessary to have some prerequisite knowledge of overtones. And, it is impossible to create sounds that you had in mind without knowing basic music theory and the fundamentals of a bass note and the overtones to some extent.

Various sounds can be created by controlling overtones, but the level of manipulation in the overtones is subtle, and the ratio of overtones to other overtones is important. Even if you know the theory, it is not easy to create sounds. In other words, it is a tricky synthesis method for anyone when the number of overtones you have to control is quite large.

Programmatic Additive Synthesis uhm language

Rather, it is more efficient to use programmatic thinking when creating sounds from an additive synthesis perspective. u-he has a scripting language for creating wavetables called uhm language. Using this language, you can flexibly control up to 1,024 harmonics. The formula below is a program that adds up to 1,024 overtones to create a sawtooth wave. What would be a difficult task by hand can be created with just one line of code.

Spectrum lowest=1 highest=1024 "1 / index"

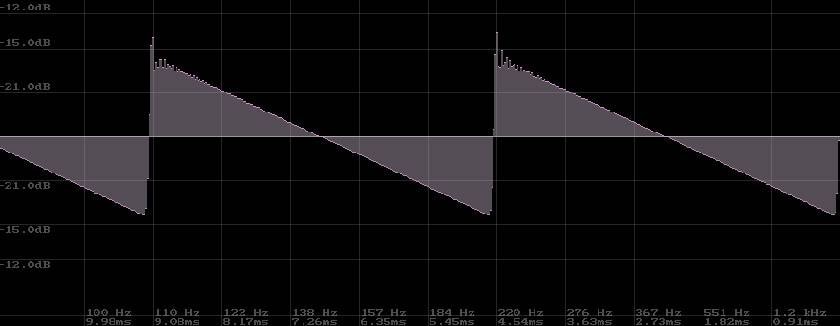
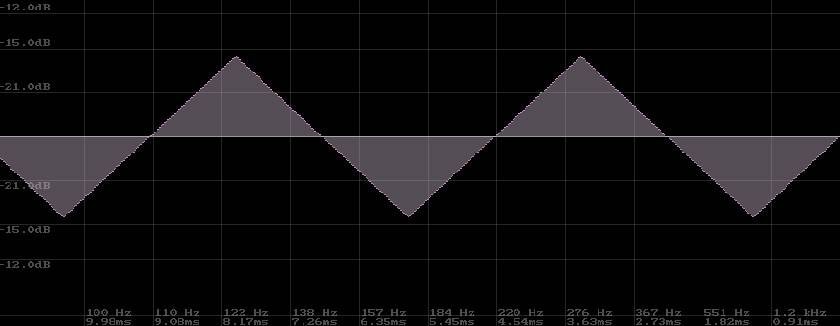
Since a triangular wave requires plus and minus, it is not realistic to create it by manipulating Zebralette's bar, but uhm can be expressed with the following formula.

Spectrum lowest=1 highest=60 "1/(index^2) * ((index % 2)==1) * (1-2*((index % 4)==3))"

The above is more about mathematics and physics than about making the sound of an instrument, so there is not much demand for it in general. Still, the more things that can be done, the more possibilities there are. When synthesizers were introduced in the first place, some acoustic and engineering knowledge was required, but increasingly specialized knowledge is needed. Therefore, the use of selecting presets has become more common. On the other hand, the movement to create and offer sounds seems to be expanding in the future.

The “sound & person” column is made up of contributions from you.
For details about contributing, click here.